ohkay
c is the y-intercept. So, you get this first as +3
The equation of the given graph reduces to y=a sinbx +3
Functions of the form f(x) = a sin x are dilation of the curve sin x parallel to the y axis.
Now the normal graph of sin(x) lies between – 1 and + 1.
In the graph we have been given that the graph lies between + 9 and -3
So the graph has been dilated 6 times. Thus a = 6.
The equation of the given graph reduces to y = 6 sin(bx) + 3
Functions of the form f(x) = sin(bx) are dilation of the curve sinx parallel to the x – axis.
This means that the period of the graph is altered.
The graph of f(x) = sin(bx) will show b cycles in 2

radians meaning that its ...
period will be given by T=

/
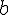
T of the graph is

b=2
The equation of the given graph reduces to y = 6 sin(2x) + 3
Comparing with the graph of y = a sin(bx) + c we get a = 6, b = 2 and c = 3.