All Pure Mathematics CIE (9709) doubts here!!!
My small Doubt :
Solve the equation 3sin2theta+4cos theta = 4, giving all the roots, in the interval
0 <= theta <= 2 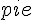
Hey man, that's not very difficult. I won't do it but i'll surely give you a hint

Let theta be x
Just replace
sin2x by
1 - cos2xThen let
cos x = y and you'll be having a quadratic formula which you solve to obtain the required solution

=> 3y2 - 4y + 1 = 0
I guess that you'll be able to complete the number yourself from now
